NCERT Solutions For Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.1
Download the free NCERT Maths Chapter 5 Solutions for Class 10 here Arithmetic Progressions in Maths Class 10 NCERT Solutions are a great resource for homework assistance. For class 10 maths 5.1 ncert solutions Expert Bhautikstudy.com Teachers developed the NCERT Solutions. Complete solutions to all the problems in NCERT Textbook’s Chapter 5 Math Class 10 Pair of Linear Equations in Two Variables Exercise 5.1.
Class 10 Maths 5.1 NCERT Solutions Question 1.
1. In which of the following situations, does the list of numbers involved make an arithmetic progression and why?
(i) The taxi fare after each km when the fare is ₹ 15 for the first km and ₹ 8 for each additional km.
(ii) The amount of air present in a cylinder when a vacuum pump removes 1/4 of the air remaining in the cylinder at a time.
(iii) The cost of digging a well after every metre of digging, when it costs ₹ 150 for the first metre and rises by ₹ 50 for each subsequent metre.
(iv) The amount of money in the account every year, when ₹ 10000 is deposited at compound interest at 8% per annum.
Solution:
Class 10 Maths 5.1 NCERT Solutions Question 2.
2. Write first four terms of the A.P. when the first term a and the common difference are given as follows:
(i) a = 10, d = 10
(ii) a = -2, d = 0
(iii) a = 4, d = – 3
(iv) a = -1 d = 1/2
(v) a = – 1.25, d = – 0.25
Solution:
Class 10 Maths 5.1 NCERT Solutions Question 3.
3. For the following A.P.s, write the first term and the common difference.
(i) 3, 1, – 1, – 3 …
(ii) -5, – 1, 3, 7 …
(iii) 1/3, 5/3, 9/3, 13/3 ….
(iv) 0.6, 1.7, 2.8, 3.9 …
Solution:
Class 10 Maths 5.1 NCERT Solutions Question 4.
4. Which of the following are APs? If they form an A.P. find the common difference d and write three more terms.
(i) 2, 4, 8, 16 …
(ii) 2, 5/2, 3, 7/2 ….
(iii) -1.2, -3.2, -5.2, -7.2 …
(iv) -10, – 6, – 2, 2 …
(v) 3, 3 + √2, 3 + 2√2, 3 + 3√2
(vi) 0.2, 0.22, 0.222, 0.2222 ….
(vii) 0, – 4, – 8, – 12 …
(viii) -1/2, -1/2, -1/2, -1/2 ….
(ix) 1, 3, 9, 27 …
(x) a, 2a, 3a, 4a …
(xi) a, a2, a3, a4 …
(xii) √2, √8, √18, √32 …
(xiii) √3, √6, √9, √12 …
(xiv) 12, 32, 52, 72 …
(xv) 12, 52, 72, 73 …
Solution:
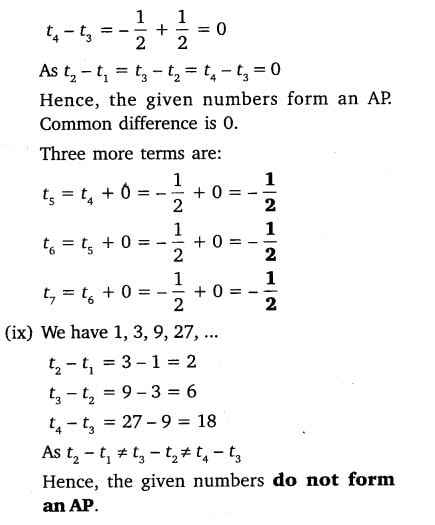
Maths in Class 10: Arithmetic Progressions
In Class 10 Maths 5.1 NCERT Solutions Students will talk about patterns in terms that come after those that come after by adding a certain number to the terms that came before in this chapter. Additionally, they see how to calculate the sum of n successive terms and the nth term. When students tackle real-world situations, they will successfully understand arithmetic progression.
The nth term and the sum of the first n terms of an A.P. are derived using the Arithmetic Progression and applied to solve real-world situations in this chapter. From the perspective of the Class 10 exam, this is one of the most significant chapters. Since practically all competitive exams include questions on arithmetic progression, understanding an arithmetic progression is a fundamental and crucial subject to learn here Class 10 Maths 5.1 NCERT Solutions play an Important role.
The composition of the Quadratic Equations Class 10 Maths 5.1 NCERT Solutions has been designed to facilitate comprehension for all students. The knowledgeable staff at Bhautik Study has worked really hard to create an engaging and enjoyable Class 10 Maths 5.1 NCERT Solutions Chapter 5 Solutions. Without a question, maths is a difficult subject to learn. The formulas are difficult for pupils to learn, and they have difficulty using tactics when they should and here Class 10 Maths 5.1 NCERT Solutions play an important role.
Arithmatic Progressions in Chapter 5 of Maths Class 10 can be used to get the value of x. Aside from this, Class 10 Maths Chapter 5 provides about three methods for this. However, not everyone can easily understand all three of these approaches. Nevertheless, the Bhautik Study professionals have presented all three approaches in a very engaging manner that any student can readily understand in Chapter 5 Maths Class 10 NCERT Solutions. The explanations of all quadratic equation principles and formulas are clear. For the purpose of providing basic knowledge, the origin of the formula, its discovery process, the person who discovered it, and several other items are initially discussed.
Subsequently, NCERT Solution Class 10 Maths Chapter 5 provides numerous solved examples to help students learn how to use formulae and answer numerical problems. The Bhautik Study specialists have completed all of the NCERT exercises for the students. For the students’ practice, Class 10 Maths 5.1 NCERT Solutions NCERT Solutions also includes a few unanswered questions. In addition, the questions from the preceding year have been included in between the NCERT Solutions. It is also stated which question appeared in which year for the convenience of the pupils. For students’ better preparation, a few mock test papers are also included at the end of the PDF.
With complete assurance, we state that the NCERT Class 10 Maths 5.1 NCERT Solutions Solutions by Bhautik Study is the only thing you need to succeed in the board exams. Students only need to click on the link on the Bhautik Study website to obtain the free PDF.
What is the Advantage of Class 10 Maths NCERT Solutions Chapter 5 Provided by Bhautik Study?
Class 10 Maths 5.1 NCERT Solutions
Arithmetic Progressions (AP) are an essential topic in mathematics, commonly taught in Class 10 (in many educational systems). Here’s a concise overview of arithmetic progressions:
Definition:
An arithmetic progression (AP) is a sequence of numbers in which the difference between consecutive terms is constant. This constant difference is called the “common difference.”
General Form:
The general form of an arithmetic progression is:
Where:
- is the first term,
- is the common difference,
- is the number of terms in the sequence.
Properties:
- Common Difference (d): The difference between any two consecutive terms is constant.
- Nth Term (or General Term): The nth term of an AP is given by: .
- Sum of ‘n’ Terms (Arithmetic Series): The sum of the first terms of an AP, denoted by , is given by:
Examples of Arithmetic Progressions:
- , .
- , .
- , .
Finding Terms and Sums:
- Finding a Term: Given the first term , the common difference , and the position , we can find the th term using the formula: .
- Finding the Sum: To find the sum of the first terms , we use the formula mentioned above.
Solving Problems:
- Finding Unknown Terms: Given some terms of an AP, you may be asked to find the unknown terms or the common difference.
- Finding Sum: Sometimes, you may need to find the sum of a certain number of terms in the AP.
- Finding Position: You might also be asked to find the position of a certain term in the AP.
Applications:
Arithmetic progressions have numerous applications in real life, such as calculating financial progressions, analyzing patterns, and in various fields of science and engineering.
Understanding arithmetic progressions is crucial not only for Class 10 mathematics but also for higher-level mathematics and various applications in different fields here Class 10 Maths 5.1 NCERT Solutions play an important role.
