Table of Contents
Toggleclass 10 maths 3.2 solutions
NCERT Solutions For Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.2
Download the free NCERT Maths Chapter 3 Solutions for Class 10 here. Pair of Linear Equations in Two Variables in Maths Class 10 NCERT Solutions are a great resource for homework assistance. For class 10 maths 3.2 solutions Expert Bhautikstudy.com Teachers developed the NCERT Solutions. Complete solutions to all the problems in NCERT Textbook’s Chapter 3 Math Class 10 Pair of Linear Equations in Two Variables Exercise 3.2
Class 10 Maths 3.2 Solutions Question 1.
1. Solve the following pair of linear equations by the substitution method
(i) x + y = 14, x – y = 4
(ii) s – t = 3,(s/3) + (t/2) = 6
(iii) 3x – y = 3, 9x – 3y = 9
(iv) 0.2x + 0.3y = 1.3, 0.4x + 0.5y = 2.3
(v) √2 x+√3 y = 0, √3 x-√8 y = 0
(vi) (3x/2) – (5y/3) = -2, (x/3) + (y/2) = (13/6)
Solution:




Class 10 Maths 3.2 Solutions Question 2.
2. Solve 2x + 3y = 11 and 2x – 4y = – 24 and hence find the value of ‘m’ for which y = mx + 3.
Solution:


Class 10 Maths 3.2 Solutions Question 3.
3. Form the pair of linear equations for the following problems and find their solution by substitution method:
(i) The difference between two numbers is 26 and one number is three times the other. Find them.
(ii) The larger of two supplementary angles exceeds the smaller by 18 degrees. Find them.
(iii) The coach of a cricket team buys 7 bats and 6 balls for ₹3800. Later, she buys 3 bats and 5 balls for ₹1750. Find the cost of each bat and each ball.
(iv) The taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of 10 km, the charge paid is ₹105 and for a journey of 15 km, the charge paid is ₹155. What are the fixed charges and the charges per km? How much does a person have to pay for travelling a distance of 25 km?
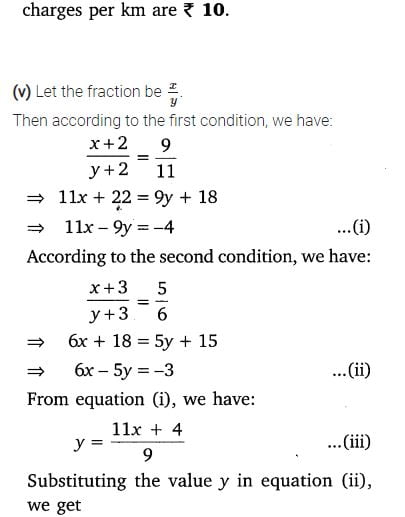
(v) A fraction becomes 9/11, if 2 is added to both the numerator and the denominator. If 3 is added to both the numerator and the denominator, it becomes 5/6. Find the fraction.
(vi) Five years hence, the age of Jacob will be three times that of his son. Five year ago, Jacob’s age was seven times that of his son. What are their present ages?
Solutions:







Maths in Class 10: Pair of Linear Equations in Two Variables
Overview
You have covered Linear Equations in Two Variables in previous sessions. It has also been studied that there are an endless number of solutions to a linear equation in two variables. This chapter will review and expand on the understanding of linear equations in two variables to include pairs of linear equations in two variables.
Two Differential Linear Equations
A linear equation in two variables, x and y, is an equation that takes the form ax + by + c = 0, where a, b, and c are real values and a and b are not zeros. A pair of values, one for x and the other for y, that equalises the two sides of the equation is the solution to such a problem. Along with appropriate examples, the topic also covers the geometrical representation of a pair of linear equations in two variables here class 10 maths 3.1 solutions play an important role.
Pair of Linear Equations in Two Variables, Class 10 Maths System of Mind Maps for Two Linear Equations in Two Variables
In class 10 maths 3.2 solutions In several disciplines, including physics, engineering, economics, and computer graphics, polynomials are widely employed.
These are basic polynomial notes appropriate for a class 10 setting. They address fundamental ideas, procedures, factorization, equations, and application domains. class 10 maths 3.2 solutions are crucial in this context.
Linear Equation Depicted in Two Variables
Each solution (x, y) to a linear equation in two variables, ax + by + c = 0, corresponds to a point on the line representing the equation, and vice versa. Every linear equation in two variables is graphically represented as a line here class 10 maths 3.2 solutions play an important role.
Procedure for Solving a Set of Two Linear Equations in Two Variables