Table of Contents
Toggleclass 10 maths 3.1 solutions
NCERT Solutions For Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.1
Download the free NCERT Maths Chapter 3 Solutions for Class 10 here. Pair of Linear Equations in Two Variables in Maths Class 10 NCERT Solutions are a great resource for homework assistance. For class 10 maths 3.1 solutions Expert Bhautikstudy.com Teachers developed the NCERT Solutions. Complete solutions to all the problems in NCERT Textbook’s Chapter 3 Math Class 10 Pair of Linear Equations in Two Variables Exercise 3.1.
Class 10 Maths 3.1 Solutions Question 1.
1. Form the pair of linear equations in the following problems, and find their solutions graphically.
(i) 10 students of Class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
(ii) 5 pencils and 7 pens together cost 50, whereas 7 pencils and 5 pens together cost 46. Find the cost of one pencil and that of one pen.
Solution:
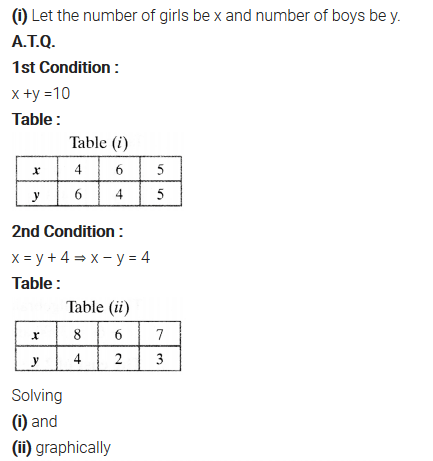
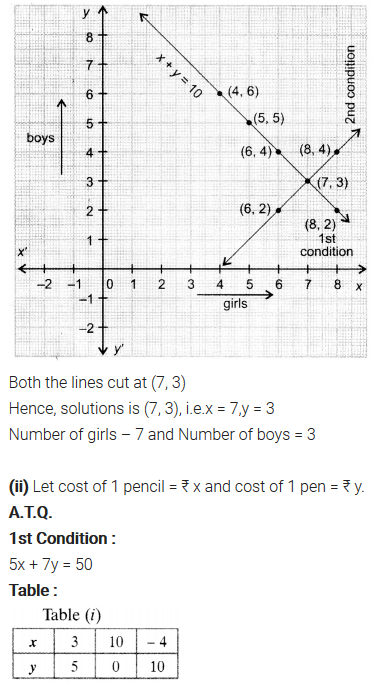

Class 10 Maths 3.1 Solutions Question 2.
2. On comparing the ratios a1/a2 , b1/b2 , c1/c2 find out whether the lines representing the following pairs of linear equations intersect at a point, are parallel or coincident:
(i) 5x – 4y + 8 = 0
7x + 6y – 9 = 0
(ii) 9x + 3y + 12 = 0
18x + 6y + 24 = 0
(iii) 6x – 3y + 10 = 0
2x – y + 9 = 0
Solution:


Class 10 Maths 3.1 Solutions Question 3.
3. On comparing the ratio, (a1/a2) , (b1/b2) , (c1/c2) find out whether the following pair of linear equations are consistent, or inconsistent.
(i) 3x + 2y = 5 ; 2x – 3y = 7
(ii) 2x – 3y = 8 ; 4x – 6y = 9
(iii)(3/2)x+(5/3)y = 7; 9x – 10y = 14
(iv) 5x – 3y = 11 ; – 10x + 6y = –22
(v)(4/3)x+2y = 8 ; 2x + 3y = 12
Solutions:


Class 10 Maths 3.1 Solutions Question 4.
4. Which of the following pairs of linear equations are consistent/inconsistent? If consistent, obtain the solution graphically:
(i) x + y = 5, 2x + 2y = 10
(ii) x – y = 8, 3x – 3y = 16
(iii) 2x + y – 6 = 0, 4x – 2y – 4 = 0
(iv) 2x – 2y – 2 = 0, 4x – 4y – 5 = 0
Solutions:



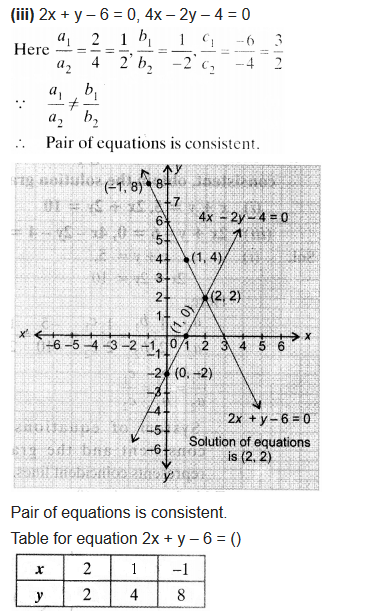

Class 10 Maths 3.1 Solutions Question 5.
5. Half the perimeter of a rectangular garden, whose length is 4 m more than its width, is 36 m. Find the dimensions of the garden.
Solutions:


Class 10 Maths 3.1 Solutions Question 6.
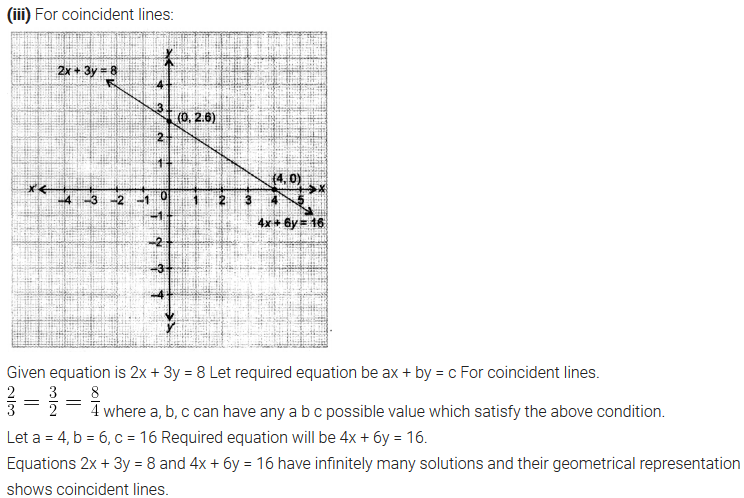
6. Given the linear equation 2x + 3y – 8 = 0, write another linear equation in two variables such that the geometrical representation of the pair so formed is:
(i) Intersecting lines
(ii) Parallel lines
(iii) Coincident lines
Solutions:

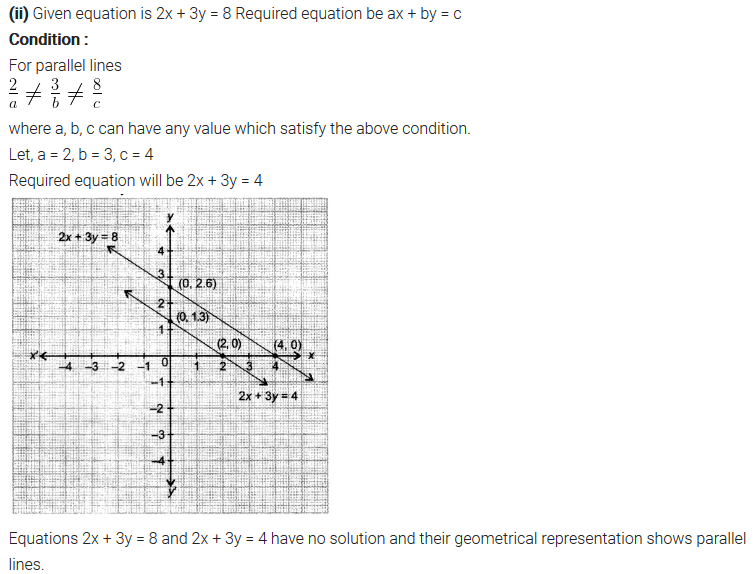
Class 10 Maths 3.1 Solutions Question 7.
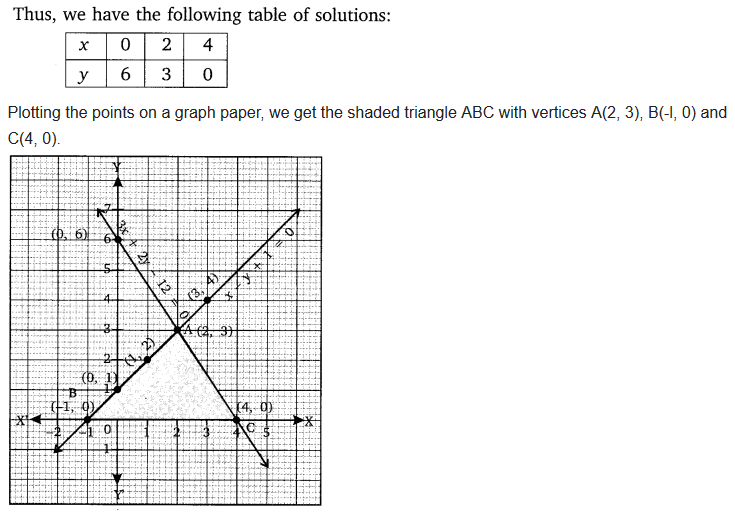
7. Draw the graphs of the equations x – y + 1 = 0 and 3x + 2y – 12 = 0. Determine the coordinates of the vertices of the triangle formed by these lines and the x-axis, and shade the triangular region.
Solutions:
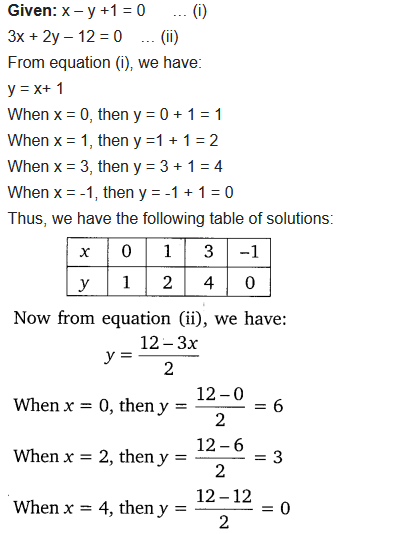
Maths in Class 10: Pair of Linear Equations in Two Variables
Overview
You have covered Linear Equations in Two Variables in previous sessions. It has also been studied that there are an endless number of solutions to a linear equation in two variables. This chapter will review and expand on the understanding of linear equations in two variables to include pairs of linear equations in two variables.
Two Differential Linear Equations
A linear equation in two variables, x and y, is an equation that takes the form ax + by + c = 0, where a, b, and c are real values and a and b are not zeros. A pair of values, one for x and the other for y, that equalises the two sides of the equation is the solution to such a problem. Along with appropriate examples, the topic also covers the geometrical representation of a pair of linear equations in two variables here class 10 maths 3.1 solutions play an important role.
Pair of Linear Equations in Two Variables, Class 10 Maths System of Mind Maps for Two Linear Equations in Two Variables
In class 10 maths 3.1 solutions In several disciplines, including physics, engineering, economics, and computer graphics, polynomials are widely employed.
These are basic polynomial notes appropriate for a class 10 setting. They address fundamental ideas, procedures, factorization, equations, and application domains. class 10 maths 3.1 solutions are crucial in this context.
Linear Equation Depicted in Two Variables
Each solution (x, y) to a linear equation in two variables, ax + by + c = 0, corresponds to a point on the line representing the equation, and vice versa. Every linear equation in two variables is graphically represented as a line.
Procedure for Solving a Set of Two Linear Equations in Two Variables
The necessary solution is the coordinate of the point (x, y) that satisfies the system of pairwise linear equations in two variables. The intersection of the two lines that represent the two equations is at this position.
Two approaches exist for solving a set of linear equations in two variables.
(1) Visual Approach: When the point used to symbolise the answer has non-integral coordinates, this method is less practical.
(2) Algebraic Method: In cases where the point used to represent the solution has non-integral coordinates, this method is more practical.
The Substitution Method, the Elimination Method, and the Cross Multiplication Method are the three further divisions of this approach.